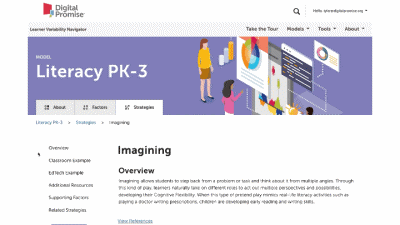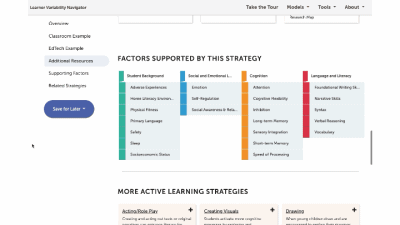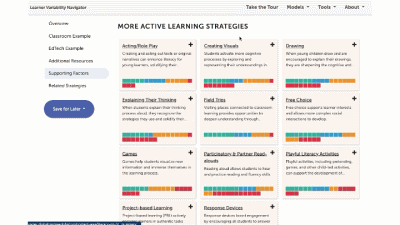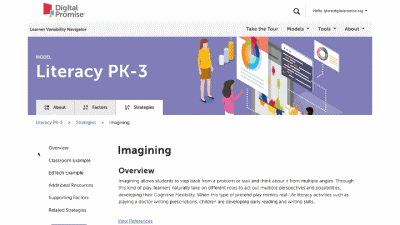
Response Devices
Overview
Response devices boost engagement by encouraging all students to answer every question. By receiving immediate, regular feedback, teachers can assess student learning and appropriately adjust instruction to meet their needs.
Example: Use This Strategy in the Classroom
Watch how this sixth grade math teacher uses Kahoot to review recent material and collect formative data on student learning. By first working through the problem individually, students can focus their Attention on solving the problem before sharing their answers anonymously with the class.
Design It into Your Product
Videos are chosen as examples of strategies in action. These choices are not endorsements of the products or evidence of use of research to develop the feature.
Learn how a cloud-based student response system, Socrative, allows for a variety of student interactions and for teachers to assess student learning. The flexibility of creating quiz questions beforehand or spontaneously and the gamified element of answering questions through space races are particularly effective features for usability and Motivation.
Additional Resources
Additional examples, research, and professional development. These resources are possible representations of this strategy, not endorsements.
Factors Supported by this Strategy
More Active Learning Strategies
Students activate more cognitive processes by exploring and representing their understandings in visual form.
Analyzing incorrect worked examples is especially beneficial for helping students develop a conceptual understanding of mathematical processes.
When students explain their thinking process aloud with guidance in response to questions or prompts, they recognize the strategies they use and solidify their understanding.
Math games allow students to practice many math skills in a fun, applied context.
Project-based learning (PBL) actively engages learners in authentic tasks designed to create products that answer a given question or solve a problem.
When students create their own number and word problems, they connect math concepts to their background knowledge and lived experiences.
Analyzing and discussing solved problems helps students develop a deeper understanding of abstract mathematical processes.
